Resistor: Difference between revisions
From DT Online
m (Added Template) |
m (Added category links) |
||
| Line 80: | Line 80: | ||
[[Category:Secondary]] | |||
[[Category:Components]] | |||
[[Category:Electronics and Control]] | |||
Revision as of 15:11, 28 April 2015
Resistors are used to control the flow of current in a circuit, by ‘resisting’ the flow of electrons.
The value of a resistor is measured in ohms (symbol: Ω). This unit is named after Georg Ohm, the German physicist and mathematician who described Ohm's Law
Resistance (R) is defined as the ratio of the potential difference (voltage) across a conductor, to the current flowing through it - ie.: Resistance (ohms) = Voltage (volts) / Current (amps)
The unit of voltage (symbol: V) is named after Alessandro Volta and the unit of current (symbol: A) is named after André-Marie Ampère
Resistors are available in values from fractions of ohms up to many millions of ohms (Megohms) although only certain ‘preferred’ values are readily available.
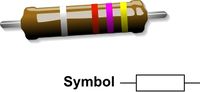
To identify the resistance value of resistors a ‘colour code’ system is often used.
The colours are shown on a series of colour bands at one end of the resistor. 2 colour bands plus a multiplier are shown but sometimes there is a 3rd colour band before the multiplier to show hundredths.
At the other end of the resistor there can also be a gold or silver band to denote the accuracy tolerance of the value displayed.
The colour code is really just an extension of the colours of the rainbow and so a similar mnemonic could be devised to help remember them - e.g. Big Brawny Richard of York Gave Battle in Very Grey Weather
Can you think of your own?
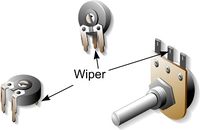
Variable resistors or potentiometers are used where the flow of current in a circuit needs to be occasionally varied such as for:
- Volume Control
- Frequency Control
- Output Voltage Control
They are available in a range of sizes.
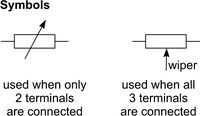
The international symbol for a variable resistor or potentiometer when drawing circuit diagrams is shown opposite
Resistors can be joined end to end in series.
In this arrangement the total resistance is equal to the value of all the resistors added together - i.e. : R(total)= R1 + R2 + R3...etc
Resistors may also be connected together in 'parallel’.
In this arrangement the total resistance is calculated as follows:
- ___1___ = _______1________
- R (Total) R1 + R2 + R3...etc
or
- R (Total) = _____R1xR2xR3_____
- R1xR2+R2xR3+R3xR1
- R (Total) = _____R1xR2xR3_____
Capacitors may also be connected together end to end in series with a resistor to create a resistor–capacitor circuit (RC circuit) in which a capacitor charges or discharges through a resistor for a known period of time known as the time constant
In this arrangement the time constant is calculated as follows:
- T(seconds)= C(farads) x R(ohms)