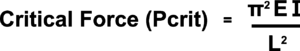Buckling: Difference between revisions
From DT Online
(Created article) |
(Added Template) |
||
| Line 1: | Line 1: | ||
[[File:ElephantPillar2.png|200px|right]] | [[File:ElephantPillar2.png|200px|right]] | ||
__TOC__ | |||
=====Description===== | |||
A '''[[Structural Member]]''' in '''[[Compression]]''' ''(i.e. a '''[[Strut]]''')'' is more likely to [https://en.wikipedia.org/wiki/Buckling '''buckle'''] first when subjected to pressure - assuming the '''[[Strut]]''' is made of a sensible material and not something so [https://en.wikipedia.org/wiki/Brittleness '''brittle'''] it is likely to explode under pressure(!) | A '''[[Structural Member]]''' in '''[[Compression]]''' ''(i.e. a '''[[Strut]]''')'' is more likely to [https://en.wikipedia.org/wiki/Buckling '''buckle'''] first when subjected to pressure - assuming the '''[[Strut]]''' is made of a sensible material and not something so [https://en.wikipedia.org/wiki/Brittleness '''brittle'''] it is likely to explode under pressure(!) | ||
::::::::::[[File:EulersBucklingEquation.png|300px|bottom]] | ::::::::::[[File:EulersBucklingEquation.png|300px|bottom]] | ||
=====Features and Applications===== | |||
The point at which a '''[[Strut|strut]]''' might [https://en.wikipedia.org/wiki/Buckling '''buckle'''] can be assessed using a simplified form of [https://en.wikipedia.org/wiki/Euler's_critical_load '''Euler's Buckling Equation'''] as follows: | The point at which a '''[[Strut|strut]]''' might [https://en.wikipedia.org/wiki/Buckling '''buckle'''] can be assessed using a simplified form of [https://en.wikipedia.org/wiki/Euler's_critical_load '''Euler's Buckling Equation'''] as follows: | ||
| Line 14: | Line 22: | ||
*'''L''' = the length of the '''[[Strut]]''' ''(this is taken as the total length if the strut is assumed to be pin-jointed at each end but would vary with other fixing methods - see [https://en.wikipedia.org/wiki/Euler's_critical_load '''Euler's Buckling Equation'''])'' | *'''L''' = the length of the '''[[Strut]]''' ''(this is taken as the total length if the strut is assumed to be pin-jointed at each end but would vary with other fixing methods - see [https://en.wikipedia.org/wiki/Euler's_critical_load '''Euler's Buckling Equation'''])'' | ||
{{Construction Kits Buyers Guide}} | |||
[[Category:Terminology]] | [[Category:Terminology]] | ||
Revision as of 19:30, 3 September 2016
Description
A Structural Member in Compression (i.e. a Strut) is more likely to buckle first when subjected to pressure - assuming the Strut is made of a sensible material and not something so brittle it is likely to explode under pressure(!)
Features and Applications
The point at which a strut might buckle can be assessed using a simplified form of Euler's Buckling Equation as follows:
Where:
- Pcrit = the Critical Force at which the strut will start to buckle.
- E = the Young's Modulus of the material (obtainable from tables or by experiment).
- Capital I = the Moment of Inertia about the Neutral Axis (for a rectangular beam this is its breadth times the depth cubed divided by 12).
- L = the length of the Strut (this is taken as the total length if the strut is assumed to be pin-jointed at each end but would vary with other fixing methods - see Euler's Buckling Equation)
|
Click top left to enlarge |