Intersecting Chords: Difference between revisions
From DT Online
mNo edit summary |
mNo edit summary |
||
| (3 intermediate revisions by the same user not shown) | |||
| Line 20: | Line 20: | ||
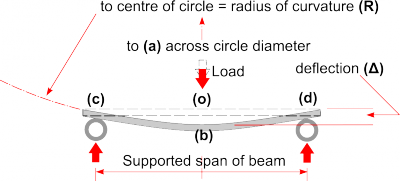
* but, for small deflections, Δ<sup>2</sup> will be negligible | * but, for small deflections, Δ<sup>2</sup> will be negligible | ||
* so '''Bending Radius ''(R)'' = cd<sup>2</sup> ÷ (8 x Δ)''' | * so '''Bending Radius ''(R)'' = cd<sup>2</sup> ÷ (8 x Δ)''' | ||
''(see | ''(see '''<dtamazon product="0306812835" type="text">Structures: Or Why Things Don't Fall Down by J.E.Gordon</dtamazon>)''''' </span> | ||
|[[File:BeamChordsTheorem.png|400px|right]] | |[[File:BeamChordsTheorem.png|400px|right]] | ||
|} | |} | ||
| Line 27: | Line 27: | ||
[[Category:Secondary]] | [[Category:Secondary]] | ||
[[Category:Terminology]] | [[Category:Terminology]] | ||
Latest revision as of 15:22, 4 July 2017
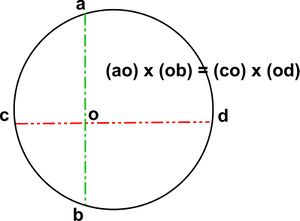
This theorem relates to a characteristic of a cyclic quadlitateral, the diagonals of which are two intersecting chords of the circumscribing circle.
The Intersecting Chords Theorem states that the relationship (ao x ob = co x od) is true for any two intersecting chords, whether or not one of them is a diameter.
| Measuring Beam Deflections |
The theorem can be useful when measuring the radius of bending of a deflected beam if one of the chords is taken to be the length of the beam e.g.
Bending Radius (R) can be calculated as follows:
(see Structures: Or Why Things Don't Fall Down by J.E.Gordon |